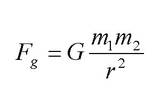Gravity – What Keeps Us Together
(Source is open content, mixed references so vast it is impossible to identify the first…)
Mathematically, the above equation translates into the force equation shown. Its blurry for a reason. We attempt to define yet at some point the definition will be re-defined, reborn, changed a little or alot, or just dimissed. For now we shall keep it there but keep it blurry…
In this equation, the quantities are defined as:
- Fg = The force of gravity (typically in newtons)
- G = The gravitational constant, which adds the proper level of proportionality to the equation. The value of G is 6.67259 x 10-11 N * m2 / kg2, although the value will change if other units are being used.
- m1 & m1 = The masses of the two particles (typically in kilograms)
- r = The straight-line distance between the two particles (typically in meters)
Interpreting the Equation
This equation gives us the magnitude of the force, which is an attractive force and therefore always directed toward the other particle. As per Newton’s Third Law of Motion, this force is always equal and opposite. Now despite their different mass and sizes, they pull on each other with equivalent force. Newton’s Three Laws of Motion give us the tools to interpret the motion caused by the force and we see that the particle with less mass (which may or may not be the smaller particle, depending upon their densities) will accelerate more than the other particle. This is why light objects fall to the Earth considerably faster than the Earth falls toward them. Still, the force acting on the light object and the Earth is of identical magnitude, even though it doesn’t look that way.
It is also significant to note that the force is inversely proportional to the square of the distance between the objects. As objects get further apart, the force of gravity drops very quickly. At most distances, only objects with very high masses such as planets, stars, galaxies, and black holes have any significant gravity effects.
Weak Interaction
The weak interaction is a very powerful force that acts on the scale of the atomic nucleus. It causes phenomena such as beta decay. It has been consolidated with electromagnetism as a single interaction called the “electroweak interaction.” The weak interaction is mediated by the W boson (there are actually two types, the W+ and W– bosons) and also the Z boson.
Strong Interaction
The strongest of the forces is the aptly named strong interaction, which is the force that, among other things, keeps nucleons (protons & neutrons) bound together. In the helium atom, for example, it is strong enough to bind two protons together even though their positive electrical charges cause them to repulse each other.
In essence, the strong interaction allows particles called gluons to bind together quarks to create the nucleons in the first place. Gluons can also interact with other gluons, which gives the strong interaction a theoretically infinite distance, although it’s major manifestations are all at the subatomic level.
Electromagnetism
Electromagnetism is the interaction of particles with an electrical charge. Charged particles at rest interact through electrostatic forces, while in motion they interact through both electrical and magnetic forces.
For a long time, the electric and magnetic forces were considered to be different forces, but they were finally unified by James Clerk Maxwell in 1864, under Maxwell’s equations. In the 1940s, quantum electrodynamics consolidated electromagnetism with quantum physics.
Electromagnetism is perhaps the most obviously prevalent force in our world, as it can affect things at a reasonable distance and with a fair amount of force.
Questions asked:
When you say simple formulae for Electromagnetism and Gravity I bet you mean the following two nice and understandable equations:
Electromagnetism – Force between two charges Q and q which are r meters apart is given by
F(em)= k*Q*q/(r*r)
Gravity – Force between two masses M and m which are r meters apart is given by F(grav)= G*M*m/(r*r) where k and G are some constants measured in the labs. I am sure, you will be surprised by my answer for the next two forces. Weak interaction – Force between two weakly interacting objects which are r meters apart is given by: F(weak) = 0 N Strong interaction – Force between two strongly interacting objects which are r meters apart is given by: F(strong) = 10000000000 N or equally well 0 N ( I picked an arbitrary number of 0, just to demonstrate that the strong force is HUGE.) Now you are probably wondering, what the heck I meant when I said the weak force is zero and the strong force is enormous (or zero).
Here is the answer:
In order to understand the above forces, I have to talk about their range. The range of the force is defined as the distance between the interacting objects, where you still feel a non-negligible force. In this sense, the ELECTROMAGNETISM and GRAVITY are LONG RANGE forces. (Just think about the big distance between the clouds and the Earth, when the lightning hits, or the distance between planets and the sun. I am sure, you will agree with me that these distances are large, so the range of the forces is long.)
On the other hand, the WEAK force is a SHORT RANGE force. Essentially, the weak force vanishes on any larger distance then the size of the nucleus!!! This means, if you take two “weakly” interacting objects, and you place them further from each of other then the radius of the nucleus, the force will be 0!!! That is why I said at the beginning that F(weak) = 0 N.
The fourth force, the STRONG force is a peculiar one. It is a SHORT range force, even tough the force between two strongly interacting objects GROWS as their distance grows!!!
Now you say, wait a minute. I just said, the interaction range is defined such that it is a distance when the force between objects is non-negligible. So if you go far enough with the two strongly interacting object, the force between them will surely grow to be big enough ( this is why I said F(strong) = 10000000000 N) to call the interaction long range. So why do I call the strong force a short range force?
Do you know why?
Because it is not possible to pull two strongly interacting object far enough without “destroying” them.
What do I mean by that?
When you have the two strongly interacting objects very close (e.g. as close as being locked in a volume of the nucleus), the force between them is very small. If you try to pull them apart, the force starts to grow! Very rapidly grow. Nature wants to keep the strongly interacting objects always together. When you pull them too far ( remember, this too far is still an extremely small distance, such as the radius of a nucleus) they pop up from the vacuum another two objects, quickly pair up with them in such a way, that the distance within the pairs will be again very small, but the distance of the two pairs can be big. (Just think about a spring. A spring has two ends. If you stretch the string too hard, it will break into two smaller strings again with two ends.)
Once more, – when you pull too hard, you break. Break means production of 2 pairs of objects. Within the pairs, strong force acts and keeps the partners together. But the pairs themselves are free to move, the force between the pairs is 0. (This is why I said above that F(strong) is equally well 0 N.)
I am sure that now you feel, that the strong interaction is again a SHORT RANGE force. On big scales we have all the strongly interacting objects paired up and the force between them is essentially 0.
Now, that I explained the fundamental difference between the forces like electromagnetism or gravity AND forces like weak or strong force, we are ready to answer your question.
The formulae for electromagnetism and gravity are nice and simple because they are valid only for r’s (remember, the r was the distance between the interacting objects) of our everyday life scales. (Everyday life deals with scales from the tenth of an inch to hundreds of thousands of miles). On these scales, the strong and the weak forces are zero.
On the other hand, on the very very small scales, (size of the nucleus) the above formulae for electromagnetism and gravity are not valid, they are much more complicated. Similarly, the weak and strong formulae are not anymore 0, but are also complicated expressions.
Conclusion: Whether some formulae are nice and simple, depends on the typical scale you are talking about. On our everyday life scales, the electromagnetism, gravity, weak and strong formulae are nice and simple. On much smaller scales, like the radius of a nucleus, the formulae are extremely complicated and not simple and nice at all for neither of the 4 mentioned forces.